research papers
Quantitative phase retrieval in X-ray Zernike phase contrast microscopy
aDepartment of Physics, University of Science and Technology of China, Hefei, Anhui 230026, People's Republic of China, bNational Synchrotron Radiation Laboratory, University of Science and Technology of China, Hefei, Anhui 230029, People's Republic of China, cInstitute of Fluid Physics, China Academy of Engineering Physics, Mianyang, Sichuan 621900, People's Republic of China, and dBeijing Synchrotron Radiation Facility, Institute of High Energy Physics, Chinese Academy of Sciences, Beijing 100049, People's Republic of China
*Correspondence e-mail: wangnsrl@ustc.edu.cn
In recent years, increasing attention has been devoted to X-ray phase contrast imaging, since it can provide high-contrast images by using phase variations. Among the different existing techniques, Zernike phase contrast microscopy is one of the most popular phase-sensitive techniques for investigating the fine structure of the sample at high spatial resolution. In X-ray Zernike phase contrast microscopy, the image contrast is indeed a mixture of absorption and phase contrast. Therefore, this technique just provides qualitative information on the object, which makes the interpretation of the image difficult. In this contribution, an approach is proposed for quantitative phase retrieval in X-ray Zernike phase contrast microscopy. By shifting the phase of the direct light by π/2 and 3π/2, two images of the same object are measured successively. The phase information of the object can then be quantitatively retrieved by a proper combination of the measured images. Numerical experiments were carried out and the results confirmed the feasibility of the proposed method. It is expected that the proposed method will find widespread applications in biology, materials science and so on.
Keywords: X-ray microscopy; Zernike phase contrast; phase retrieval; phase ring.
1. Introduction
The unique characteristics of X-rays are short wavelength and high penetration, and therefore X-ray microscopy is especially well suited to high-resolution tomographic imaging of several-micrometre-thick biological specimens (Kirz et al., 1995![Kirz, J., Jacobsen, C. & Howells, M. (1995). Q. Rev. Biophys. 28, 33-130. [Kirz, J., Jacobsen, C. & Howells, M. (1995). Q. Rev. Biophys. 28, 33-130.]](../../../../../../logos/arrows/s_arr.gif) ). However, when imaging biological or other samples made of light elements with hard X-rays, the absorption contrast is always too weak (Henke et al., 1993
). However, when imaging biological or other samples made of light elements with hard X-rays, the absorption contrast is always too weak (Henke et al., 1993![Henke, B. L., Gullikson, E. M. & Davis, J. C. (1993). At. Data Nucl. Data Tables, 54, 181-342. [Henke, B. L., Gullikson, E. M. & Davis, J. C. (1993). At. Data Nucl. Data Tables, 54, 181-342.]](../../../../../../logos/arrows/s_arr.gif) ). Considering that the interaction of light with an object causes wavefront distortions, F. Zernike developed phase contrast microscopy (Zernike, 1942
). Considering that the interaction of light with an object causes wavefront distortions, F. Zernike developed phase contrast microscopy (Zernike, 1942![Zernike, F. (1942). Physica, 9, 974-986. [Zernike, F. (1942). Physica, 9, 974-986.]](../../../../../../logos/arrows/s_arr.gif) ). This technique was then proven to be powerful for the observation of weak phase objects with the visible microscope. The phase contrast imaging method has also been successfully applied to X-ray microscopy (Schmahl et al., 1995
). This technique was then proven to be powerful for the observation of weak phase objects with the visible microscope. The phase contrast imaging method has also been successfully applied to X-ray microscopy (Schmahl et al., 1995![Schmahl, G., Rudolph, D., Guttmann, P., Schneider, G., Thieme, J. & Niemann, B. (1995). Rev. Sci. Instrum. 66, 1282-1286. [Schmahl, G., Rudolph, D., Guttmann, P., Schneider, G., Thieme, J. & Niemann, B. (1995). Rev. Sci. Instrum. 66, 1282-1286.]](../../../../../../logos/arrows/s_arr.gif) ; Holzner et al., 2010
; Holzner et al., 2010![Holzner, C., Feser, M., Vogt, S., Hornberger, B., Baines, S. B. & Jacobsen, C. (2010). Nat. Phys. 6, 883-887. [Holzner, C., Feser, M., Vogt, S., Hornberger, B., Baines, S. B. & Jacobsen, C. (2010). Nat. Phys. 6, 883-887.]](../../../../../../logos/arrows/s_arr.gif) ; Stampanoni et al., 2010
; Stampanoni et al., 2010![Stampanoni, M., Mokso, R., Marone, F., Vila-Comamala, J., Gorelick, S., Trtik, P., Jefimovs, K. & David, C. (2010). Phys. Rev. B, 81, 140105. [Stampanoni, M., Mokso, R., Marone, F., Vila-Comamala, J., Gorelick, S., Trtik, P., Jefimovs, K. & David, C. (2010). Phys. Rev. B, 81, 140105.]](../../../../../../logos/arrows/s_arr.gif) ; Wang et al., 2014
; Wang et al., 2014![Wang, D., Li, N., Wang, Z., Gao, K., Zhang, Y., Luo, Y., Wang, S., Bao, Y., Shao, Q. & Wu, Z. (2014). J. Synchrotron Rad. 21, 1175-1179. [Wang, D., Li, N., Wang, Z., Gao, K., Zhang, Y., Luo, Y., Wang, S., Bao, Y., Shao, Q. & Wu, Z. (2014). J. Synchrotron Rad. 21, 1175-1179.]](../../../../../../logos/arrows/s_arr.gif) ). Following the development of high-quality Fresnel zone plates in the soft X-ray energy region, X-ray Zernike phase contrast imaging was first reported in 1995 (Schmahl et al., 1995
). Following the development of high-quality Fresnel zone plates in the soft X-ray energy region, X-ray Zernike phase contrast imaging was first reported in 1995 (Schmahl et al., 1995![Schmahl, G., Rudolph, D., Guttmann, P., Schneider, G., Thieme, J. & Niemann, B. (1995). Rev. Sci. Instrum. 66, 1282-1286. [Schmahl, G., Rudolph, D., Guttmann, P., Schneider, G., Thieme, J. & Niemann, B. (1995). Rev. Sci. Instrum. 66, 1282-1286.]](../../../../../../logos/arrows/s_arr.gif) ).
).
In the soft X-ray region, a zone-plate-based transmission X-ray microscope has achieved a spatial resolution of 12 nm (Chao et al., 2009![Chao, W., Kim, J., Rekawa, S., Fischer, P. & Anderson, E. H. (2009). Opt. Express, 17, 17669-17677. [Chao, W., Kim, J., Rekawa, S., Fischer, P. & Anderson, E. H. (2009). Opt. Express, 17, 17669-17677.]](../../../../../../logos/arrows/s_arr.gif) ), and the advantage of using absorption information has already been successfully demonstrated in the `water window' soft X-ray region (McDermott et al., 2009
), and the advantage of using absorption information has already been successfully demonstrated in the `water window' soft X-ray region (McDermott et al., 2009![McDermott, G., Le Gros, M. A., Knoechel, C. G., Uchida, M. & Larabell, C. A. (2009). Trends Cell Biol. 19, 587-595. [McDermott, G., Le Gros, M. A., Knoechel, C. G., Uchida, M. & Larabell, C. A. (2009). Trends Cell Biol. 19, 587-595.]](../../../../../../logos/arrows/s_arr.gif) ; Larabell & Nugent, 2010
; Larabell & Nugent, 2010![Larabell, C. A. & Nugent, K. A. (2010). Curr. Opin. Struct. Biol. 20, 623-631. [Larabell, C. A. & Nugent, K. A. (2010). Curr. Opin. Struct. Biol. 20, 623-631.]](../../../../../../logos/arrows/s_arr.gif) ; Hanssen et al., 2011
; Hanssen et al., 2011![Hanssen, E., Knoechel, C., Klonis, N., Abu-Bakar, N., Deed, S., LeGros, M., Larabell, C. & Tilley, L. (2011). J. Struct. Biol. 173, 161-168. [Hanssen, E., Knoechel, C., Klonis, N., Abu-Bakar, N., Deed, S., LeGros, M., Larabell, C. & Tilley, L. (2011). J. Struct. Biol. 173, 161-168.]](../../../../../../logos/arrows/s_arr.gif) ). However, the limited penetration of soft X-rays hinders widespread applications of soft X-ray microscopy. To overcome this limitation, the research activity of X-ray Zernike phase contrast microscopy has been shifted to the intermediate energy and hard X-ray region (Awaji et al., 2002
). However, the limited penetration of soft X-rays hinders widespread applications of soft X-ray microscopy. To overcome this limitation, the research activity of X-ray Zernike phase contrast microscopy has been shifted to the intermediate energy and hard X-ray region (Awaji et al., 2002![Awaji, M., Suzuki, Y., Takeuchi, A., Takano, H., Kamijo, N., Tamura, S. & Yasumoto, M. (2002). J. Synchrotron Rad. 9, 125-127. [Awaji, M., Suzuki, Y., Takeuchi, A., Takano, H., Kamijo, N., Tamura, S. & Yasumoto, M. (2002). J. Synchrotron Rad. 9, 125-127.]](../../../../../../logos/arrows/s_arr.gif) ; Tkachuk et al., 2007
; Tkachuk et al., 2007![Tkachuk, A., Duewer, F., Cui, H., Feser, M., Wang, S. & Yun, W. (2007). Z. Kristallogr. 222, 650-655. [Tkachuk, A., Duewer, F., Cui, H., Feser, M., Wang, S. & Yun, W. (2007). Z. Kristallogr. 222, 650-655.]](../../../../../../logos/arrows/s_arr.gif) ; Yin et al., 2007
; Yin et al., 2007![Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118. [Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118.]](../../../../../../logos/arrows/s_arr.gif) ; Liu et al., 2011
; Liu et al., 2011![Liu, Y., Andrews, J. C., Wang, J., Meirer, F., Zhu, P., Wu, Z. & Pianetta, P. (2011). Opt. Express, 19, 540-545. [Liu, Y., Andrews, J. C., Wang, J., Meirer, F., Zhu, P., Wu, Z. & Pianetta, P. (2011). Opt. Express, 19, 540-545.]](../../../../../../logos/arrows/s_arr.gif) ; Yuan et al., 2012
; Yuan et al., 2012![Yuan, Q., Zhang, K., Hong, Y., Huang, W., Gao, K., Wang, Z., Zhu, P., Gelb, J., Tkachuk, A., Hornberger, B., Feser, M., Yun, W. & Wu, Z. (2012). J. Synchrotron Rad. 19, 1021-1028. [Yuan, Q., Zhang, K., Hong, Y., Huang, W., Gao, K., Wang, Z., Zhu, P., Gelb, J., Tkachuk, A., Hornberger, B., Feser, M., Yun, W. & Wu, Z. (2012). J. Synchrotron Rad. 19, 1021-1028.]](../../../../../../logos/arrows/s_arr.gif) ; Wang et al., 2013
; Wang et al., 2013![Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392. [Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392.]](../../../../../../logos/arrows/s_arr.gif) ; Vartiainen et al., 2014a
; Vartiainen et al., 2014a![Vartiainen, I., Warmer, M., Goeries, D., Herker, E., Reimer, R., David, C. & Meents, A. (2014a). J. Synchrotron Rad. 21, 790-794. [Vartiainen, I., Warmer, M., Goeries, D., Herker, E., Reimer, R., David, C. & Meents, A. (2014a). J. Synchrotron Rad. 21, 790-794.]](../../../../../../logos/arrows/s_arr.gif) ,b
,b![Vartiainen, I., Mokso, R., Stampanoni, M. & David, C. (2014b). Opt. Lett. 39, 1601-1604. [Vartiainen, I., Mokso, R., Stampanoni, M. & David, C. (2014b). Opt. Lett. 39, 1601-1604.]](../../../../../../logos/arrows/s_arr.gif) ; Yang et al., 2014
; Yang et al., 2014![Yang, Y., Heine, R., Cheng, Y., Wang, C. C., Song, Y. F. & Baumbach, T. (2014). Appl. Phys. Lett. 105, 094101. [Yang, Y., Heine, R., Cheng, Y., Wang, C. C., Song, Y. F. & Baumbach, T. (2014). Appl. Phys. Lett. 105, 094101.]](../../../../../../logos/arrows/s_arr.gif) ). This trend is partly due to the fact that, within these energy regimes, and for biomedical samples, the real part of the complex which describes the phase shift property, is always much larger than the imaginary part describing the absorption property. Moreover, the ratio between the real and imaginary part increases with the incident photon energy (Henke et al., 1993
). This trend is partly due to the fact that, within these energy regimes, and for biomedical samples, the real part of the complex which describes the phase shift property, is always much larger than the imaginary part describing the absorption property. Moreover, the ratio between the real and imaginary part increases with the incident photon energy (Henke et al., 1993![Henke, B. L., Gullikson, E. M. & Davis, J. C. (1993). At. Data Nucl. Data Tables, 54, 181-342. [Henke, B. L., Gullikson, E. M. & Davis, J. C. (1993). At. Data Nucl. Data Tables, 54, 181-342.]](../../../../../../logos/arrows/s_arr.gif) ). So it is obvious that the phase contrast is more conspicuous in the intermediate and hard X-ray region. As recently reported, in the intermediate X-ray range, a Zernike phase contrast microscope can provide good image contrast for biological samples (Wang et al., 2013
). So it is obvious that the phase contrast is more conspicuous in the intermediate and hard X-ray region. As recently reported, in the intermediate X-ray range, a Zernike phase contrast microscope can provide good image contrast for biological samples (Wang et al., 2013![Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392. [Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392.]](../../../../../../logos/arrows/s_arr.gif) ).
).
For an X-ray microscope operated in the Zernike phase contrast mode, the measured image intensity contains a mixture of absorption and phase information. As a consequence, the interpretation of the image and the quantitative tomographic reconstruction require a quantitative retrieval of the phase information from intensity measurements in X-ray Zernike phase contrast microscopy. Quantitative phase retrieval in a transmission X-ray microscope was reported for the first time by Yin et al. They put forward a combination of the transport of intensity equation (Teague, 1983![Teague, M. R. (1983). J. Opt. Soc. Am. 73, 1434-1441. [Teague, M. R. (1983). J. Opt. Soc. Am. 73, 1434-1441.]](../../../../../../logos/arrows/s_arr.gif) ) and the self-consistent wave propagation method (Allen et al., 2004
) and the self-consistent wave propagation method (Allen et al., 2004![Allen, L. J., McBride, W., O'Leary, N. L. & Oxley, M. P. (2004). Ultramicroscopy, 100, 91-104. [Allen, L. J., McBride, W., O'Leary, N. L. & Oxley, M. P. (2004). Ultramicroscopy, 100, 91-104.]](../../../../../../logos/arrows/s_arr.gif) ) to extract quantitative phase information of the sample (Yin et al., 2007
) to extract quantitative phase information of the sample (Yin et al., 2007![Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118. [Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118.]](../../../../../../logos/arrows/s_arr.gif) ). But this method required a series of sample intensity images, where multiple exposures could induce serious radiation damage to biological samples. Working with a transmission X-ray microscope, Liu et al. (2011
). But this method required a series of sample intensity images, where multiple exposures could induce serious radiation damage to biological samples. Working with a transmission X-ray microscope, Liu et al. (2011![Liu, Y., Andrews, J. C., Wang, J., Meirer, F., Zhu, P., Wu, Z. & Pianetta, P. (2011). Opt. Express, 19, 540-545. [Liu, Y., Andrews, J. C., Wang, J., Meirer, F., Zhu, P., Wu, Z. & Pianetta, P. (2011). Opt. Express, 19, 540-545.]](../../../../../../logos/arrows/s_arr.gif) ) introduced a new quantitative phase retrieval method, where a series of the sample's intensity images were measured by changing the energy of the incident X-rays. Several exposures were also required in this approach.
) introduced a new quantitative phase retrieval method, where a series of the sample's intensity images were measured by changing the energy of the incident X-rays. Several exposures were also required in this approach.
In this contribution, we propose an approach for quantitative phase retrieval in X-ray Zernike phase contrast microscopy, which takes advantage of the quantitative phase measurements in optical microscopy (Fiolka et al., 2009![Fiolka, R., Wicker, K., Heintzmann, R. & Stemmer, A. (2009). Opt. Express, 17, 12407-12417. [Fiolka, R., Wicker, K., Heintzmann, R. & Stemmer, A. (2009). Opt. Express, 17, 12407-12417.]](../../../../../../logos/arrows/s_arr.gif) ; Gao et al., 2011
; Gao et al., 2011![Gao, P., Yao, B., Harder, I., Lindlein, N. & Torcal-Milla, F. J. (2011). Opt. Lett. 36, 4305-4307. [Gao, P., Yao, B., Harder, I., Lindlein, N. & Torcal-Milla, F. J. (2011). Opt. Lett. 36, 4305-4307.]](../../../../../../logos/arrows/s_arr.gif) ). The key point of this method is the use of two different phase rings. With the two phase rings put in the beam successively, two intensity measurements are recorded. By a proper combination of the two intensity images, we can retrieve quantitative phase information of the investigated sample. Numerical experiments are carried out, and the results confirm the validity of the proposed method.
). The key point of this method is the use of two different phase rings. With the two phase rings put in the beam successively, two intensity measurements are recorded. By a proper combination of the two intensity images, we can retrieve quantitative phase information of the investigated sample. Numerical experiments are carried out, and the results confirm the validity of the proposed method.
2. Methods
A schematic diagram of X-ray Zernike phase contrast microscopy is shown in Fig. 1![[link]](../../../../../../logos/arrows/s_arr.gif) . Typically, a capillary reflective condenser produces hollow cone illumination, which is designed to nearly match the numerical aperture of the zone plate objective. Then, the light illuminating the sample is divided into a diffracted and an undiffracted part. The undiffracted light (i.e. direct light) passes through the phase ring, which is placed at the back focal plane of the zone plate objective, and then interferes with the diffracted light in the detector plane. Finally, the phase modulations of the sample are translated into intensity modulations recorded by the detector.
. Typically, a capillary reflective condenser produces hollow cone illumination, which is designed to nearly match the numerical aperture of the zone plate objective. Then, the light illuminating the sample is divided into a diffracted and an undiffracted part. The undiffracted light (i.e. direct light) passes through the phase ring, which is placed at the back focal plane of the zone plate objective, and then interferes with the diffracted light in the detector plane. Finally, the phase modulations of the sample are translated into intensity modulations recorded by the detector.
![[Figure 1]](pp5067fig1thm.gif) | Figure 1 Schematic setup of X-ray Zernike phase contrast microscopy. |
In the following, we derive the approach for quantitative phase retrieval in a transmission X-ray microscope operated in Zernike phase contrast mode. The interactions of X-rays with matter can be described by the complex n = ![[1 - \delta - i\beta]](teximages/pp5067fi1.gif) , where the real part
, where the real part ![[\delta]](teximages/pp5067fi2.gif) represents the phase shift property and the imaginary part
represents the phase shift property and the imaginary part ![[\beta]](teximages/pp5067fi3.gif) the absorption of the material.
the absorption of the material.
Consider a model object where a feature of interest is embedded in its homogeneous background. Assuming monochromatic plane wave illumination of unit amplitude, the complex amplitude Af traveling through the particular feature is (Rudolph et al., 1990![Rudolph, D., Schmahl, G. & Niemann, B. (1990). Amplitude and Phase Contrast in X-ray Microscopy. New York: Plenum. [Rudolph, D., Schmahl, G. & Niemann, B. (1990). Amplitude and Phase Contrast in X-ray Microscopy. New York: Plenum.]](../../../../../../logos/arrows/s_arr.gif) ; Wang et al., 2013
; Wang et al., 2013![Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392. [Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392.]](../../../../../../logos/arrows/s_arr.gif) )
)
where ![[\lambda]](teximages/pp5067fi5.gif) is the X-ray wavelength,
is the X-ray wavelength, ![[{\delta_{\rm{f}}}]](teximages/pp5067fi6.gif) and
and ![[{\beta_{\rm{f}}}]](teximages/pp5067fi7.gif) are the real and imaginary parts of the of the feature, respectively,
are the real and imaginary parts of the of the feature, respectively, ![[{\delta_{\rm{b}}}]](teximages/pp5067fi8.gif) and
and ![[{\beta_{\rm{b}}}]](teximages/pp5067fi9.gif) are the real and imaginary parts of the of the background material, respectively, and D and t denote the thickness of the background and the feature, respectively. The complex amplitude Ab traveling through the homogeneous background is given by
are the real and imaginary parts of the of the background material, respectively, and D and t denote the thickness of the background and the feature, respectively. The complex amplitude Ab traveling through the homogeneous background is given by
The insertion of the feature generates diffraction, and the amplitude of the diffracted wave can be approximated by Ad = Af - Ab.
In Zernike phase contrast mode, the wave Ab passes through the phase ring with a thickness tp, the complex amplitude transmission of which is given by
where ![[{\delta_{\rm{p}}}]](teximages/pp5067fi15.gif) and
and ![[{\beta_{\rm{p}}}]](teximages/pp5067fi16.gif) represent the real and imaginary parts of the of the phase ring material, respectively. To obtain Zernike phase contrast, the thickness of the phase ring is designed to introduce a phase shift of the undiffracted light by π/2 (positive phase contrast) or 3π/2 (negative phase contrast). Herein, we assume that the modulation transfer function of the detector is 100% for all spatial frequencies. Then, the amplitude of the feature image can be written as
represent the real and imaginary parts of the of the phase ring material, respectively. To obtain Zernike phase contrast, the thickness of the phase ring is designed to introduce a phase shift of the undiffracted light by π/2 (positive phase contrast) or 3π/2 (negative phase contrast). Herein, we assume that the modulation transfer function of the detector is 100% for all spatial frequencies. Then, the amplitude of the feature image can be written as
and the corresponding intensity is given by
In the absence of a feature, the surroundings' intensity is given by
So the normalized sample's intensity image is given by
In the proposed phase retrieval method, we use two different phase rings. One is for the positive phase contrast and the other for the negative phase contrast. In the positive phase contrast mode the thickness of the phase ring satisfies ![[{{2\pi{\delta_{\rm{p}}}{t_{\rm{p1}}}}/\lambda}]](teximages/pp5067fi17.gif) =
= ![[{\pi / 2}]](teximages/pp5067fi18.gif) , and one has Tp1 =
, and one has Tp1 = ![[i\exp ({{{ - 2\pi {\beta_{\rm{p}}}{t_{\rm{p1}}}} /\lambda}} )]](teximages/pp5067fi20.gif) . Then the amplitude of the feature image is given by
. Then the amplitude of the feature image is given by
with a1 = ![[\exp({{{-2\pi{\beta_{\rm{p}}}{t_{\rm{p1}}}}/\lambda}}).]](teximages/pp5067fi22.gif) The image intensities with and without the feature are given by
The image intensities with and without the feature are given by
respectively, where ![[\varphi]](teximages/pp5067fi23.gif) =
= ![[{{2\pi({{\delta_{\rm{f}}}-{\delta_{\rm{b}}}})t}/\lambda}]](teximages/pp5067fi24.gif) . We obtain the normalized intensity image,
. We obtain the normalized intensity image,
In transmission X-ray microscopy, the typical feature size is of the order of tens of nanometres. Therefore we can reasonably apply the first-order Taylor expansion to (11)![[link]](../../../../../../logos/arrows/s_arr.gif) ,
,
Under the above approximation, the normalized intensity image has the following expression, and a detailed derivation from equations (11)![[link]](../../../../../../logos/arrows/s_arr.gif) to (13)
to (13)![[link]](../../../../../../logos/arrows/s_arr.gif) can be found in Appendix A
can be found in Appendix A![[link]](../../../../../../logos/arrows/s_arr.gif) ,
,
In the negative phase contrast mode, a second phase ring is utilized. The thickness is chosen to introduce a ![[3\pi/2]](teximages/pp5067fi25.gif) phase shift onto the undiffracted beam. Following a procedure similar to that used for the positive phase contrast mode, we obtain the corresponding normalized image intensity,
phase shift onto the undiffracted beam. Following a procedure similar to that used for the positive phase contrast mode, we obtain the corresponding normalized image intensity,
with a2 = ![[\exp({{{-2\pi{\beta_{\rm{p}}}{t_{\rm{p2}}}}/\lambda}})]](teximages/pp5067fi27.gif) . By a proper combination of equations (13)
. By a proper combination of equations (13)![[link]](../../../../../../logos/arrows/s_arr.gif) and (14), we can retrieve the phase shift of the feature given by
and (14), we can retrieve the phase shift of the feature given by
where B = ![[{({a_1^2{{I^{\,\prime}_1}} - a_2^2{{I^{\,\prime}_2}} - a_1^2 + a_2^2})}/]](teximages/pp5067fi28.gif)
![[\{a_1a_2[a_1I^{\,\prime}_1+a_2I^{\,\prime}_2]](teximages/pp5067fi29.gif) − (a1+a2)]}.
− (a1+a2)]}.
A detailed derivation of equations (15)![[link]](../../../../../../logos/arrows/s_arr.gif) and (16)
and (16)![[link]](../../../../../../logos/arrows/s_arr.gif) can be found in Appendix B
can be found in Appendix B![[link]](../../../../../../logos/arrows/s_arr.gif) . Equation (16)
. Equation (16)![[link]](../../../../../../logos/arrows/s_arr.gif) represents the main result of this contribution, i.e. the phase retrieval method. By use of two intensity measurements with two different phase rings, we can retrieve the phase shift of the sample quantitatively. Note that in the derivation of (16)
represents the main result of this contribution, i.e. the phase retrieval method. By use of two intensity measurements with two different phase rings, we can retrieve the phase shift of the sample quantitatively. Note that in the derivation of (16)![[link]](../../../../../../logos/arrows/s_arr.gif) we have used the linear approximation given in equation (12)
we have used the linear approximation given in equation (12)![[link]](../../../../../../logos/arrows/s_arr.gif) . When the linear approximation does not hold well, the retrieval result by use of (16)
. When the linear approximation does not hold well, the retrieval result by use of (16)![[link]](../../../../../../logos/arrows/s_arr.gif) will deviate from the theoretical true values. To validate the proposed method, we numerically simulated an X-ray Zernike phase contrast microscope and performed phase retrieval using measured image intensities.
will deviate from the theoretical true values. To validate the proposed method, we numerically simulated an X-ray Zernike phase contrast microscope and performed phase retrieval using measured image intensities.
3. Results and discussion
Numerical experiments are performed to demonstrate the feasibility of the proposed method. We simulated an X-ray Zernike phase contrast microscope with a photon energy of 2.5 keV (Wang et al., 2013![Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392. [Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387-392.]](../../../../../../logos/arrows/s_arr.gif) ). A Fresnel zone plate (400 nm-thick Au, 30 nm outmost zone width) was used as the objective to reach a spatial resolution better than 30 nm. The microscope used two phase rings made of nickel; one was π/2-phase shifting and the other was 3π/2-phase shifting. In our simulation, Poisson-distributed shot noise is considered in the measured images.
). A Fresnel zone plate (400 nm-thick Au, 30 nm outmost zone width) was used as the objective to reach a spatial resolution better than 30 nm. The microscope used two phase rings made of nickel; one was π/2-phase shifting and the other was 3π/2-phase shifting. In our simulation, Poisson-distributed shot noise is considered in the measured images.
Two test samples are constructed for both quantitative measurement and evaluation. The of the test samples are found from the Index of Refraction data (https://henke.lbl.gov/optical_constants/getdb2.html). The first test sample is a plastic zone plate made of polymethyl methacrylate (PMMA) on silicon nitride (Yin et al., 2007![Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118. [Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118.]](../../../../../../logos/arrows/s_arr.gif) ). This sample acts as a representative of a weakly absorbing object. The thicknesses were set to 1000 nm, 800 nm, 600 nm, 400 nm, 200 nm and 200 nm from the inner to the outer rings, to test the feasibility of the proposed method in the case of distinct phase shifts.
). This sample acts as a representative of a weakly absorbing object. The thicknesses were set to 1000 nm, 800 nm, 600 nm, 400 nm, 200 nm and 200 nm from the inner to the outer rings, to test the feasibility of the proposed method in the case of distinct phase shifts.
The results of the first sample are shown in Fig. 2![[link]](../../../../../../logos/arrows/s_arr.gif) . Figs. 2(a) and 2(b)
. Figs. 2(a) and 2(b)![[link]](../../../../../../logos/arrows/s_arr.gif) present the simulated intensity images for a π/2 phase ring and a 3π/2 phase ring, respectively. In these two images, Poisson-distributed shot noise is added. As expected, contrast reversal appears owing to the different phase shifts. With these two images as input, we retrieved the phase shift of the sample by use of equations (15)
present the simulated intensity images for a π/2 phase ring and a 3π/2 phase ring, respectively. In these two images, Poisson-distributed shot noise is added. As expected, contrast reversal appears owing to the different phase shifts. With these two images as input, we retrieved the phase shift of the sample by use of equations (15)![[link]](../../../../../../logos/arrows/s_arr.gif) and (16)
and (16)![[link]](../../../../../../logos/arrows/s_arr.gif) , and the result is shown in Fig. 2(c)
, and the result is shown in Fig. 2(c)![[link]](../../../../../../logos/arrows/s_arr.gif) . For comparison, Fig. 2(d)
. For comparison, Fig. 2(d)![[link]](../../../../../../logos/arrows/s_arr.gif) displays the true phase shift of the sample. As can be seen, Figs. 2(c) and 2(d)
displays the true phase shift of the sample. As can be seen, Figs. 2(c) and 2(d)![[link]](../../../../../../logos/arrows/s_arr.gif) match well qualitatively. Furthermore, a line profile comparison is performed. As shown in Fig. 3
match well qualitatively. Furthermore, a line profile comparison is performed. As shown in Fig. 3![[link]](../../../../../../logos/arrows/s_arr.gif) , a quantitative agreement is achieved. It is noted that a phase shift as large as 0.55 rad can be retrieved precisely. The small mismatch in the region of the outer ring can be attributed to the added shot noise. The results shown in Figs. 2
, a quantitative agreement is achieved. It is noted that a phase shift as large as 0.55 rad can be retrieved precisely. The small mismatch in the region of the outer ring can be attributed to the added shot noise. The results shown in Figs. 2![[link]](../../../../../../logos/arrows/s_arr.gif) and 3
and 3![[link]](../../../../../../logos/arrows/s_arr.gif) confirm the feasibility of the proposed method in the case of weak absorption objects.
confirm the feasibility of the proposed method in the case of weak absorption objects.
![[Figure 2]](pp5067fig2thm.gif) | Figure 2 (a), (b) Intensity images of the sample with a π/2 phase ring and a 3π/2 phase ring, respectively. (c), (d) Retrieved phase shift and the true phase shift of the sample, respectively. |
![[Figure 3]](pp5067fig3thm.gif) | Figure 3 Line profile comparison between the true and retrieved phase shift of the sample. |
The second test sample is a Siemens star-like sample made of copper, which is surrounded by water with a thickness of 5 µm. This sample represents a high-absorption feature in a cellular environment. The thickness of the copper ranged from 30 nm to 105 nm in steps of 5 nm arranged anticlockwise in a fan of angle π/16.
The results of the second sample are shown in Fig. 4![[link]](../../../../../../logos/arrows/s_arr.gif) . The simulated intensity images are shown in Figs. 4(a) and 4(b)
. The simulated intensity images are shown in Figs. 4(a) and 4(b)![[link]](../../../../../../logos/arrows/s_arr.gif) for π/2 and 3π/2 phase rings, respectively. Again, one can see the reversed contrast in these two images. By use of equation (16)
for π/2 and 3π/2 phase rings, respectively. Again, one can see the reversed contrast in these two images. By use of equation (16)![[link]](../../../../../../logos/arrows/s_arr.gif) , the sample phase is retrieved, and the result is presented in Fig. 4
, the sample phase is retrieved, and the result is presented in Fig. 4![[link]](../../../../../../logos/arrows/s_arr.gif) (c). For comparison, Fig. 4(d)
(c). For comparison, Fig. 4(d)![[link]](../../../../../../logos/arrows/s_arr.gif) shows the true phase information of the sample. Note that Figs. 4(c) and 4(d)
shows the true phase information of the sample. Note that Figs. 4(c) and 4(d)![[link]](../../../../../../logos/arrows/s_arr.gif) are visually in good agreement. Furthermore, Fig. 5
are visually in good agreement. Furthermore, Fig. 5![[link]](../../../../../../logos/arrows/s_arr.gif) shows the line profile for a quantitative comparison. As can be seen, the retrieval result is seriously contaminated by the added shot noise when the thickness is less than 35 nm. With a thickness range from 40 nm to 80 nm, the retrieved phase shift is an excellent match with the true value. This again confirms the effectiveness of the proposed method. With further increasing thickness, the phase shift is overestimated by use of equation (16)
shows the line profile for a quantitative comparison. As can be seen, the retrieval result is seriously contaminated by the added shot noise when the thickness is less than 35 nm. With a thickness range from 40 nm to 80 nm, the retrieved phase shift is an excellent match with the true value. This again confirms the effectiveness of the proposed method. With further increasing thickness, the phase shift is overestimated by use of equation (16)![[link]](../../../../../../logos/arrows/s_arr.gif) , and the error becomes greater. This can be explained by the violation of the validity of the first-order approximation used in equation (12)
, and the error becomes greater. This can be explained by the violation of the validity of the first-order approximation used in equation (12)![[link]](../../../../../../logos/arrows/s_arr.gif) . With a 50 nm thickness, the intensity transmission is 0.9492, which can be well approximated by 0.9478. However, when the thickness is increased to 100 nm, the transmission decreases to 0.9009. The linear approximation does not hold well, which then results in an error in the retrieved phase shift. In experiments, one can estimate the absorption of the investigated object before imaging, and then use this knowledge to modify the retrieved phase shift to be correct. Furthermore, we would like to mention that in the case of a 10% X-ray attenuation, the resulting absorption contrast is already sufficient for imaging purposes.
. With a 50 nm thickness, the intensity transmission is 0.9492, which can be well approximated by 0.9478. However, when the thickness is increased to 100 nm, the transmission decreases to 0.9009. The linear approximation does not hold well, which then results in an error in the retrieved phase shift. In experiments, one can estimate the absorption of the investigated object before imaging, and then use this knowledge to modify the retrieved phase shift to be correct. Furthermore, we would like to mention that in the case of a 10% X-ray attenuation, the resulting absorption contrast is already sufficient for imaging purposes.
![[Figure 4]](pp5067fig4thm.gif) | Figure 4 (a), (b) Intensity images of a copper Siemens-like star sample with a π/2 and a 3π/2 phase ring, respectively. (c), (d) Retrieved phase shift and the true phase information of the sample, respectively. The thickness of the copper features increases in an anticlockwise direction from 30 nm at the 3 o'clock position to 105 nm in 5 nm steps. |
![[Figure 5]](pp5067fig5thm.gif) | Figure 5 Profile comparison between the true and retrieved phase shift of the second sample. The numbers on the figure (30, 50, 70 and 90) indicate the thickness of the respective features in the sample in nanometers. |
4. Conclusion
In conclusion, we have presented a quantitative phase retrieval method in X-ray Zernike phase contrast microscopy. With the use of two distinct phase rings, two sample images are measured successively. The phase shift information can then be quantitatively retrieved by properly dealing with the two intensity measurements. Numerical experiments are performed for the cases of both weak and strong absorption objects. For these weakly absorbing objects, a good agreement is achieved between the retrieval results and theoretical true values, thus confirming the feasibility of the proposed method. However, for those objects of strong absorption, some discrepancies are observed between the retrieved results and the true values. This can be attributed to the violation of the used approximation, and may be corrected by use of prior knowledge. With the further development of X-ray optics and technologies, the proposed method may find widespread implementations at synchrotron radiation facilities.
APPENDIX A
Detailed mathematic derivation from equation (11)![[link]](../../../../../../logos/arrows/s_arr.gif) to equation (13)
to equation (13)![[link]](../../../../../../logos/arrows/s_arr.gif)
Combine equation (11)![[link]](../../../../../../logos/arrows/s_arr.gif) with equation (12)
with equation (12)![[link]](../../../../../../logos/arrows/s_arr.gif) , and equation (13)
, and equation (13)![[link]](../../../../../../logos/arrows/s_arr.gif) is calculated by
is calculated by
Similarly, equation (14)![[link]](../../../../../../logos/arrows/s_arr.gif) is calculated by
is calculated by
So the normalized intensity image with a π/2 phase ring and 3π/2 phase ring can be given by equations (13)![[link]](../../../../../../logos/arrows/s_arr.gif) and (14)
and (14)![[link]](../../../../../../logos/arrows/s_arr.gif) , respectively.
, respectively.
APPENDIX B
Detailed mathematic derivation of equations (15)![[link]](../../../../../../logos/arrows/s_arr.gif) and (16)
and (16)![[link]](../../../../../../logos/arrows/s_arr.gif)
According to the formula a12[(17)] - a22[(18)], we obtain the expression
According to the formula: a12a2[(17)] + a1a22[(18)], we obtain the following expression,
and equation (21)![[link]](../../../../../../logos/arrows/s_arr.gif) is equivalent to
is equivalent to
By calculating the ratio [(20)![[link]](../../../../../../logos/arrows/s_arr.gif) ]/[(22)
]/[(22)![[link]](../../../../../../logos/arrows/s_arr.gif) ], equation (15)
], equation (15)![[link]](../../../../../../logos/arrows/s_arr.gif) is given by
is given by
and equation (23)![[link]](../../../../../../logos/arrows/s_arr.gif) is equivalent to
is equivalent to
where B = ![[{{a_1^2{{I^{\,\prime}_1}} - a_2^2{{I^{\,\prime}_2}} - a_1^2 + a_2^2}/{\{{{a_1}{a_2}[{{a_1}{{I^{\,\prime}_1}} + {a_2}{{I^{\,\prime}_2}} - ({{a_1} + a})}]}\}}}]](teximages/pp5067fi33.gif) , and equation (24)
, and equation (24)![[link]](../../../../../../logos/arrows/s_arr.gif) is equal to equation (15)
is equal to equation (15)![[link]](../../../../../../logos/arrows/s_arr.gif) .
.
In the following, equation (24)![[link]](../../../../../../logos/arrows/s_arr.gif) is replaced by
is replaced by
With the use of the relationship in the trigonometrically function ![[{\sin^2}\varphi]](teximages/pp5067fi34.gif) +
+ ![[{\cos^2}\varphi]](teximages/pp5067fi35.gif) = 1, one obtains
= 1, one obtains
Footnotes
‡Deceased.
Acknowledgements
This work was supported by the States Key Project for Fundamental Research (2012CB825801), the National Natural Science Foundation of China (11205157, 11475170), and Anhui Provincial Natural Science Foundation (1508085 MA20).
References
 Allen, L. J., McBride, W., O'Leary, N. L. & Oxley, M. P. (2004). Ultramicroscopy, 100, 91–104. Web of Science CrossRef PubMed CAS Google Scholar
Allen, L. J., McBride, W., O'Leary, N. L. & Oxley, M. P. (2004). Ultramicroscopy, 100, 91–104. Web of Science CrossRef PubMed CAS Google Scholar
 Awaji, M., Suzuki, Y., Takeuchi, A., Takano, H., Kamijo, N., Tamura, S. & Yasumoto, M. (2002). J. Synchrotron Rad. 9, 125–127. Web of Science CrossRef CAS IUCr Journals Google Scholar
Awaji, M., Suzuki, Y., Takeuchi, A., Takano, H., Kamijo, N., Tamura, S. & Yasumoto, M. (2002). J. Synchrotron Rad. 9, 125–127. Web of Science CrossRef CAS IUCr Journals Google Scholar
 Chao, W., Kim, J., Rekawa, S., Fischer, P. & Anderson, E. H. (2009). Opt. Express, 17, 17669–17677. Web of Science CrossRef PubMed CAS Google Scholar
Chao, W., Kim, J., Rekawa, S., Fischer, P. & Anderson, E. H. (2009). Opt. Express, 17, 17669–17677. Web of Science CrossRef PubMed CAS Google Scholar
 Fiolka, R., Wicker, K., Heintzmann, R. & Stemmer, A. (2009). Opt. Express, 17, 12407–12417. Web of Science CrossRef PubMed CAS Google Scholar
Fiolka, R., Wicker, K., Heintzmann, R. & Stemmer, A. (2009). Opt. Express, 17, 12407–12417. Web of Science CrossRef PubMed CAS Google Scholar
 Gao, P., Yao, B., Harder, I., Lindlein, N. & Torcal-Milla, F. J. (2011). Opt. Lett. 36, 4305–4307. CrossRef CAS PubMed Google Scholar
Gao, P., Yao, B., Harder, I., Lindlein, N. & Torcal-Milla, F. J. (2011). Opt. Lett. 36, 4305–4307. CrossRef CAS PubMed Google Scholar
 Hanssen, E., Knoechel, C., Klonis, N., Abu-Bakar, N., Deed, S., LeGros, M., Larabell, C. & Tilley, L. (2011). J. Struct. Biol. 173, 161–168. Web of Science CrossRef CAS PubMed Google Scholar
Hanssen, E., Knoechel, C., Klonis, N., Abu-Bakar, N., Deed, S., LeGros, M., Larabell, C. & Tilley, L. (2011). J. Struct. Biol. 173, 161–168. Web of Science CrossRef CAS PubMed Google Scholar
 Henke, B. L., Gullikson, E. M. & Davis, J. C. (1993). At. Data Nucl. Data Tables, 54, 181–342. CrossRef CAS Web of Science Google Scholar
Henke, B. L., Gullikson, E. M. & Davis, J. C. (1993). At. Data Nucl. Data Tables, 54, 181–342. CrossRef CAS Web of Science Google Scholar
 Holzner, C., Feser, M., Vogt, S., Hornberger, B., Baines, S. B. & Jacobsen, C. (2010). Nat. Phys. 6, 883–887. Web of Science CrossRef CAS PubMed Google Scholar
Holzner, C., Feser, M., Vogt, S., Hornberger, B., Baines, S. B. & Jacobsen, C. (2010). Nat. Phys. 6, 883–887. Web of Science CrossRef CAS PubMed Google Scholar
 Kirz, J., Jacobsen, C. & Howells, M. (1995). Q. Rev. Biophys. 28, 33–130. CrossRef CAS PubMed Web of Science Google Scholar
Kirz, J., Jacobsen, C. & Howells, M. (1995). Q. Rev. Biophys. 28, 33–130. CrossRef CAS PubMed Web of Science Google Scholar
 Larabell, C. A. & Nugent, K. A. (2010). Curr. Opin. Struct. Biol. 20, 623–631. Web of Science CrossRef CAS PubMed Google Scholar
Larabell, C. A. & Nugent, K. A. (2010). Curr. Opin. Struct. Biol. 20, 623–631. Web of Science CrossRef CAS PubMed Google Scholar
 Liu, Y., Andrews, J. C., Wang, J., Meirer, F., Zhu, P., Wu, Z. & Pianetta, P. (2011). Opt. Express, 19, 540–545. Web of Science CrossRef PubMed Google Scholar
Liu, Y., Andrews, J. C., Wang, J., Meirer, F., Zhu, P., Wu, Z. & Pianetta, P. (2011). Opt. Express, 19, 540–545. Web of Science CrossRef PubMed Google Scholar
 McDermott, G., Le Gros, M. A., Knoechel, C. G., Uchida, M. & Larabell, C. A. (2009). Trends Cell Biol. 19, 587–595. Web of Science CrossRef PubMed CAS Google Scholar
McDermott, G., Le Gros, M. A., Knoechel, C. G., Uchida, M. & Larabell, C. A. (2009). Trends Cell Biol. 19, 587–595. Web of Science CrossRef PubMed CAS Google Scholar
 Rudolph, D., Schmahl, G. & Niemann, B. (1990). Amplitude and Phase Contrast in X-ray Microscopy. New York: Plenum. Google Scholar
Rudolph, D., Schmahl, G. & Niemann, B. (1990). Amplitude and Phase Contrast in X-ray Microscopy. New York: Plenum. Google Scholar
 Schmahl, G., Rudolph, D., Guttmann, P., Schneider, G., Thieme, J. & Niemann, B. (1995). Rev. Sci. Instrum. 66, 1282–1286. CrossRef CAS Web of Science Google Scholar
Schmahl, G., Rudolph, D., Guttmann, P., Schneider, G., Thieme, J. & Niemann, B. (1995). Rev. Sci. Instrum. 66, 1282–1286. CrossRef CAS Web of Science Google Scholar
 Stampanoni, M., Mokso, R., Marone, F., Vila-Comamala, J., Gorelick, S., Trtik, P., Jefimovs, K. & David, C. (2010). Phys. Rev. B, 81, 140105. Web of Science CrossRef Google Scholar
Stampanoni, M., Mokso, R., Marone, F., Vila-Comamala, J., Gorelick, S., Trtik, P., Jefimovs, K. & David, C. (2010). Phys. Rev. B, 81, 140105. Web of Science CrossRef Google Scholar
 Teague, M. R. (1983). J. Opt. Soc. Am. 73, 1434–1441. Google Scholar
Teague, M. R. (1983). J. Opt. Soc. Am. 73, 1434–1441. Google Scholar
 Tkachuk, A., Duewer, F., Cui, H., Feser, M., Wang, S. & Yun, W. (2007). Z. Kristallogr. 222, 650–655. Web of Science CrossRef CAS Google Scholar
Tkachuk, A., Duewer, F., Cui, H., Feser, M., Wang, S. & Yun, W. (2007). Z. Kristallogr. 222, 650–655. Web of Science CrossRef CAS Google Scholar
 Vartiainen, I., Mokso, R., Stampanoni, M. & David, C. (2014b). Opt. Lett. 39, 1601–1604. Web of Science CrossRef PubMed Google Scholar
Vartiainen, I., Mokso, R., Stampanoni, M. & David, C. (2014b). Opt. Lett. 39, 1601–1604. Web of Science CrossRef PubMed Google Scholar
 Vartiainen, I., Warmer, M., Goeries, D., Herker, E., Reimer, R., David, C. & Meents, A. (2014a). J. Synchrotron Rad. 21, 790–794. Web of Science CrossRef IUCr Journals Google Scholar
Vartiainen, I., Warmer, M., Goeries, D., Herker, E., Reimer, R., David, C. & Meents, A. (2014a). J. Synchrotron Rad. 21, 790–794. Web of Science CrossRef IUCr Journals Google Scholar
 Wang, D., Li, N., Wang, Z., Gao, K., Zhang, Y., Luo, Y., Wang, S., Bao, Y., Shao, Q. & Wu, Z. (2014). J. Synchrotron Rad. 21, 1175–1179. Web of Science CrossRef CAS IUCr Journals Google Scholar
Wang, D., Li, N., Wang, Z., Gao, K., Zhang, Y., Luo, Y., Wang, S., Bao, Y., Shao, Q. & Wu, Z. (2014). J. Synchrotron Rad. 21, 1175–1179. Web of Science CrossRef CAS IUCr Journals Google Scholar
 Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387–392. Web of Science CrossRef CAS PubMed Google Scholar
Wang, Z., Gao, K., Chen, J., Hong, Y., Ge, X., Wang, D., Pan, Z., Zhu, P., Yun, W., Jacobsen, C. & Wu, Z. (2013). Biotechnol. Adv. 31, 387–392. Web of Science CrossRef CAS PubMed Google Scholar
 Yang, Y., Heine, R., Cheng, Y., Wang, C. C., Song, Y. F. & Baumbach, T. (2014). Appl. Phys. Lett. 105, 094101. Web of Science CrossRef Google Scholar
Yang, Y., Heine, R., Cheng, Y., Wang, C. C., Song, Y. F. & Baumbach, T. (2014). Appl. Phys. Lett. 105, 094101. Web of Science CrossRef Google Scholar
 Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118. Web of Science CrossRef Google Scholar
Yin, G. C., Chen, F. R., Hwu, Y., Shieh, H. D. & Liang, K. S. (2007). Appl. Phys. Lett. 90, 181118. Web of Science CrossRef Google Scholar
 Yuan, Q., Zhang, K., Hong, Y., Huang, W., Gao, K., Wang, Z., Zhu, P., Gelb, J., Tkachuk, A., Hornberger, B., Feser, M., Yun, W. & Wu, Z. (2012). J. Synchrotron Rad. 19, 1021–1028. Web of Science CrossRef CAS IUCr Journals Google Scholar
Yuan, Q., Zhang, K., Hong, Y., Huang, W., Gao, K., Wang, Z., Zhu, P., Gelb, J., Tkachuk, A., Hornberger, B., Feser, M., Yun, W. & Wu, Z. (2012). J. Synchrotron Rad. 19, 1021–1028. Web of Science CrossRef CAS IUCr Journals Google Scholar
 Zernike, F. (1942). Physica, 9, 974–986. CrossRef Google Scholar
Zernike, F. (1942). Physica, 9, 974–986. CrossRef Google Scholar
© International Union of Crystallography. Prior permission is not required to reproduce short quotations, tables and figures from this article, provided the original authors and source are cited. For more information, click here.


 journal menu
journal menu
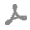






![[\eqalignno{{A_{\rm{f}}} = {}& \exp \left({{{ - 2\pi {\beta_{\rm{f}}}t} / \lambda }} \right)\exp \left({{{i2\pi {\delta_{\rm{f}}}t} / \lambda }} \right) \cr&\times\exp \left [{{{ - 2\pi {\beta_{\rm{b}}}\left({{D} - t} \right)} / \lambda }} \right] \exp\left [{{{i2\pi {\delta_{\rm{b}}}\left({D - t} \right)} / \lambda }} \right],&(1)}]](teximages/pp5067fd1.gif)
![[\eqalignno{{A_{\rm{b}}} = {}& \exp \left({{{ - 2\pi {\beta_{\rm{b}}}t} / \lambda }} \right)\exp \left({{{i2\pi {\delta_{\rm{b}}}t} / \lambda }} \right) \cr&\times \exp \left [{{{ - 2\pi {\beta_{\rm{b}}}\left({{D} - t} \right)} / \lambda }} \right] \exp\left [{{{i2\pi {\delta_{\rm{b}}}\left({D - t} \right)} / \lambda }} \right].&(2)}]](teximages/pp5067fd2.gif)
![[{T_{\rm{p}}} = \exp \left({{{ - 2\pi {\beta_{\rm{p}}}{t_{\rm{p}}}} / \lambda }} \right)\exp \left({{{i2\pi {\delta_{\rm{p}}}{t_{\rm{p}}}} /\lambda }} \right),\eqno(3)]](teximages/pp5067fd3.gif)
![[A = {A_{\rm{b}}}{T_{\rm{p}}} + {A_{\rm{d}}}\eqno(4)]](teximages/pp5067fd4.gif)
![[I = {A^*}A.\eqno(5)]](teximages/pp5067fd5.gif)
![[{I_{\rm{b}}} = A_{\rm{b}}^*T_{\rm{p}}^*{A_{\rm{b}}}{T_{\rm{p}}}.\eqno(6)]](teximages/pp5067fd6.gif)
![[I^{\,\prime} = {I / {{I_{\rm{b}}}}}.\eqno(7)]](teximages/pp5067fd7.gif)
![[\eqalignno{ {A_1} &= {A_{\rm{b}}}{T_{\rm{p1}}} + {A_{\rm{d}}} \cr & = \exp \left [{{{ - 2\pi {\beta_{\rm{b}}}\left({{D} - t} \right)} / \lambda }} \right] \exp\left [{{{i2\pi {\delta_{\rm{b}}}\left({{D} - t} \right)} / \lambda }} \right] \cr& \quad \times \Big\{ i\left[a_1\exp(-2\pi\beta_{\rm{b}}t/\lambda)\exp\left(i2\pi\delta_{\rm{b}}t/\lambda\right)\right] \cr& \quad+ \exp\left(-2\pi\beta_{\rm{f}}t/\lambda\right) \exp\left(i2\pi\delta_{\rm{f}}t/\lambda\right) \cr&\quad- \exp(-2\pi\beta_{\rm{b}}t/\lambda) \exp\left(i2\pi\delta_{\rm{b}}t/\lambda\right) \Big\}, &(8)}]](teximages/pp5067fd8.gif)
![[\eqalignno{ {I_1} = {}& A_1^*{A_1} = \exp\left({{{-4\pi{\beta_{\rm{b}}}D}/\lambda}}\right) \Big\{ a_1^2 + 1 + \exp\left [{{{4\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \cr& + 2\left({{a_1}\sin\varphi-\cos\varphi}\right) \exp\left[{{{2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}} \right] \Big\} ,&(9)}]](teximages/pp5067fd9.gif)
![[\eqalignno{{I_{b1}}&= A_{\rm{b}}^*T_{\rm{p1}}^*{A_{\rm{b}}}{T_{\rm{p1}}} \cr& = \exp\left({{{-4\pi{\beta_{\rm{p}}}{t_{\rm{p1}}}}/\lambda}}\right) \exp\left({{{-4\pi{\beta_{\rm{b}}}{D}}/\lambda }}\right) \cr& = a_1^2\exp\left({{{-4\pi{\beta_{\rm{b}}}{D}}/\lambda }} \right),&(10)}]](teximages/pp5067fd10.gif)
![[\eqalignno{ {I^{\,\prime}_1}= {}& {{{I_1}}\over{{I_{b1}}}} = {1\over{a_1^2}} \Big\{ a_1^2 + 1 + \exp\left[{{{4\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \cr&+ 2\left({{a_1}\sin\varphi - \cos\varphi } \right)\exp \left [{{{2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}}\right)t}/\lambda}}\right] \Big\}. &(11)}]](teximages/pp5067fd11.gif)
![[\eqalign{ & \exp\left [{{{4\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \approx 1 + {{4\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda, } \cr& \exp\left [{{{2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \approx 1 + {{2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda. } \cr} \eqno(12)]](teximages/pp5067fd12.gif)
![[I^{\,\prime}_1 \approx \left\{ a_1^2+2\left(1+a_1\sin\varphi-\cos\varphi\right) \left[1+2\pi\left(\beta_{\rm{b}}-\beta_{\rm{f}}\right)t/\lambda\right]\right\}/a_1^2.\eqno(13)]](teximages/pp5067fd13.gif)
![[I^{\,\prime}_2 \approx \Big\{a_2^2+2\left(1-a_2\sin\varphi-\cos\varphi\right) \left[1+2\pi\left(\beta_{\rm{b}}-\beta_{\rm{f}}\right)t/\lambda\right]\Big\}/a_2^2,\eqno(14)]](teximages/pp5067fd14.gif)
![[{{\sin \varphi }/{\left({1 - \cos \varphi } \right)}} = B,\eqno(15)]](teximages/pp5067fd15.gif)
![[\varphi = \arcsin \left [{{{2B}/{\left({{B^2} + 1} \right)}}} \right],\eqno(16)]](teximages/pp5067fd16.gif)
![[\eqalignno{ {{I^{\,\prime}_1}} & \approx {1\over{a_1^2}} \Big\{ a_1^2+1+ \left[1+4\pi\left(\beta_{\rm{b}}-\beta_{\rm{f}}\right)t/\lambda\right] \cr& \quad + 2\left(a_1\sin\varphi-\cos\varphi\right)\left[{{{1 + 2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}}\right)t}/\lambda}}\right] \Big\} \cr& = {1\over{a_1^2}} \Big\{ a_1^2+2\left[{{{1+2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right] \cr&\quad + 2\left({{a_1}\sin\varphi-\cos\varphi}\right) \left[{{{1+2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}} \right]\Big\} \cr& = {1 \over {a_1^2}}\left\{ {a_1^2 + 2\left({1 + {a_1}\sin\varphi - \cos\varphi } \right)\left [{{{1 + 2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right]} \right\}. \cr&&(17)}]](teximages/pp5067fd17.gif)
![[\eqalignno{ {{I^{\,\prime}_2}}={{{I_2}}\over{{I_{b2}}}}&= {1\over{a_2^2}} \Big\{ a_2^2+1+\exp\left[{{{4\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right] \cr& \quad +2\left({-{a_2}\sin\varphi-\cos\varphi}\right) \exp\left[{{{2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right] \Big\} \cr& \approx {1\over{a_2^2}} \Big\{ a_2^2 + 1 + \left [{{{1 + 4\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \cr&\quad + 2\left({ - {a_2}\sin\varphi -\cos\varphi } \right)\left [{{{1 + 2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \Big\} \cr& ={1 \over {a_2^2}} \Big\{a_2^2 + 2\left [{{{1 + 2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \cr&\quad + 2\left({ - {a_2}\sin\varphi -\cos\varphi } \right)\left [{{{1 + 2\pi \left({{\beta_{\rm{b}}} - {\beta_{\rm{f}}}} \right)t}/\lambda }} \right] \Big\} \cr& ={1\over{a_2^2}} \Big\{ a_2^2+ 2\left({1 - {a_2}\sin\varphi-\cos\varphi}\right) \cr& \quad\times \left[{{{1+2\pi\left({{\beta_{\rm{b}}}- {\beta_{\rm{f}}}}\right)t}/\lambda}} \right] \Big\}.&(18)}]](teximages/pp5067fd18.gif)
![[a_1^2{I^{\,\prime}_1} - a_2^2{I^{\,\prime}_2} = a_1^2 - a_2^2 + 2\left({{a_1}+{a_2}}\right) \left[{1+{{2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right]\sin\varphi, \eqno(19)]](teximages/pp5067fd19.gif)
![[\left[{1+{{2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right] \sin\varphi = {{a_1^2{{I^{\,\prime}_1}} - a_2^2 {{I^{\,\prime}_2}} - a_1^2+a_2^2} \over {2\left({{a_1}+{a_2}}\right)}}. \eqno(20)]](teximages/pp5067fd20.gif)
![[\eqalignno{ a_1^2{a_2}{{I^{\,\prime}_1}}+{a_1}a_2^2{{I^{\,\prime}_2}}&= \Big\{ a_1^2{a_2}+2{a_2}\left({1+{a_1}\sin\varphi-\cos\varphi}\right) \cr&\quad\times\left[{{{1+2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right]\Big\} \cr&\quad + \Big\{ {a_1}a_2^2+2{a_1} \left({1-{a_2}\sin\varphi-\cos\varphi}\right)\cr&\quad\times \left[{{{1+2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t}/\lambda}}\right]\Big\} \cr& = a_1^2{a_2}+{a_1}a_2^2+ 2\left({{a_1}+{a_2}}\right) \left({1-\cos\varphi}\right) \cr& \quad\times\left[{1+{{2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}}\right)t} /\lambda}}\right],&(21)}]](teximages/pp5067fd21.gif)
![[\eqalignno{ \left[{1+{{2\pi\left({{\beta_{\rm{b}}}-{\beta_{\rm{f}}}} \right)t}/\lambda}}\right] & \left({1-\cos\varphi}\right) \cr& = {{a_1^2{a_2}{{I^{\,\prime}_1}} + {a_1}a_2^2{{I^{\,\prime}_2}} - \left({a_1^2{a_2} + {a_1}a_2^2} \right)} \over {2\left({{a_1} + {a_2}} \right)}} \cr& = {{{a_1}{a_2}\left [{{a_1}{{I^{\,\prime}_1}} + {a_2}{{I^{\,\prime}_2}} - \left({{a_1} + a} \right)} \right]} \over {2\left({{a_1} + {a_2}} \right)}}.&(22)}]](teximages/pp5067fd22.gif)
![[{{\sin\varphi}\over{\left({1-\cos\varphi}\right)}} = {{a_1^2{{I^{\,\prime}_1}} - a_2^2{{I^{\,\prime}_2}} - a_1^2 + a_2^2} \over {{a_1}{a_2}\left [{{a_1}{{I^{\,\prime}_1}} + {a_2}{{I^{\,\prime}_2}} - \left({{a_1} + a} \right)} \right]}},\eqno(23)]](teximages/pp5067fd23.gif)
![[{{\sin\varphi}\over{\left({1-\cos\varphi}\right)}}=B,\eqno(24)]](teximages/pp5067fd24.gif)
![[\cos\varphi= {{B-\sin\varphi}\over{B}}.\eqno(25)]](teximages/pp5067fd25.gif)
![[\eqalign{ & \sin^2\varphi+\cos^2\varphi = \sin^2\varphi+\left({{B-\sin\varphi}\over{B}}\right)^2 = 1 \cr& \Rightarrow {{{B^2}\sin^2\varphi + {B^2} - 2B\sin \varphi + {{\sin }^2}\varphi } \over {{B^2}}} = 1 \cr& \Rightarrow \sin \varphi = {{2B} \over {{B^2} + 1}}_{\vphantom{\Big|}} \cr& \Rightarrow \varphi = \arcsin \left [{{{2B} / {\left({{B^2} + 1} \right)}}} \right],}\eqno(26)]](teximages/pp5067fd26.gif)